Physics - Motion - Motion Along a Straight Line

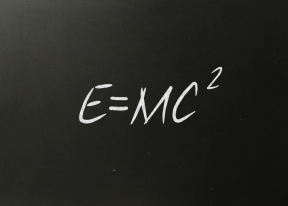
AVERAGE VELOCITY
Imagine a car is moving along a straight roadway. To observe the car's motion, we need a coordinate system. The path which the car is moving is called the x-axis. The point at which the car begins motion is the origin, or O. We choose the point furthest along the x-axis in the positive direction on the car to represent the point at which the car lays on the x-axis. We can call this a particle.
An informative way to observe the car's motion is the change in the particle's coordinate x over a time interval, t. Suppose that 1 second after the particle is at O, the particle is 15 meters away from O. The displacement of the particle is a vector that points from O to the ending location, P2 (as in point 2). This vector lays on the x-axis. The x-component of the displacement is the change in the value of x (15 meters - 0 meters) = 15 meters, that took place during the time interval of 1 second. We define the car's average velocity during this time interval as a "vector quantity" whose x component is the change in x divided by the time interval. In this case our average velocity is 15 meters / 1 second = 15 m/s.

INSTANTANEOUS VELOCITY
To find instantaneous velocity of the car at P1 (point 1), we move P2 closer and closer to P1 and compute the average velocity over shorter and shorter distances and time intervals. Both values become small but the ratio does not necessarily. In other terms, the limit of the change in distance over the change in time as time approaches zero is called the derivative of x with respect to t.
Since t is always positive, the sign of the instantaneous velocity depends on the x component.

AVERAGE ACCELERATION
Again consider a car moving along the x-axis and the furthest point on the car in the positive x direction as a "particle." Suppose that at time t1 (first measurement of time) the particle is at point P1 and has x-component of instantaneous velocity V1x. At a later time t2, the car is at P2 and has x-component of velocity V2x. The x-component of velocity change by the amount V2x - V1x. During the time interval t2 - t1.
Average acceleration of the particle as it moving from P1 to P2 is a vector quantity whose x-component Aav-x (or average X-acceleration in a straight line) equals the change in the x-component of velocity, divided by the time interval.

INSTANTANEOUS ACCELERATION
Similar to instantaneous velocity, instantaneous acceleration is the limit of the average acceleration as the time interval approaches zero. OR, instantaneous acceleration equals the derivative of velocity with time.